Enoncé de l'exercice n° 8-1 : (Extrait : n°4 page 167 livre 2nde Hachette Education)
Dans un repère orthonormé, on considère les points suivants :
| \(\displaystyle A\left(-5;8\right)\) | \(\displaystyle B\left(6;5\right)\) | \(\displaystyle C\left(-1;3\right)\) | \(\displaystyle D\left(3;2\right)\) | \(\displaystyle E\left(7;-7\right)\) |
Question 1 : Les points \(\displaystyle A\), \(\displaystyle C\) et \(\displaystyle E\) sont-ils alignés ?
Question 2 : Les droites \(\displaystyle \left(AB\right)\) et \(\displaystyle \left(CD\right)\) sont-elles parallèles ?
Envoyer la proposition de travail
Lancer l'interactivité
Enoncé de l'exercice n° 8-2 : (Extrait : n°5 page 167 livre 2nde Hachette Education)
Dans un repère orthonormé, on considère les points suivants :
| \(\displaystyle A\left(2;6\right)\) | \(\displaystyle B\left(-3;5\right)\) | \(\displaystyle C\left(4;1\right)\) | \(\displaystyle D\left(-8;-1\right)\) | \(\displaystyle E\left(6;-4\right)\) |
Question 1 : Les points \(\displaystyle A\), \(\displaystyle C\) et \(\displaystyle E\) sont-ils alignés ?
Question 2 : Les droites \(\displaystyle \left(AB\right)\) et \(\displaystyle \left(CD\right)\) sont-elles parallèles ?
Question 3 : Le triangle \(\displaystyle BCD\) est-il isocèle ?
Envoyer la proposition de travail
Lancer l'interactivité
On considère les points \(\displaystyle A\left(2;3\right)\) , \(\displaystyle B\left(1;-1\right)\) et \(\displaystyle C\left(6;2\right)\)
Question 1 : Démontrer que le triangle \(\displaystyle ABC\) est rectangle et isocèle.
Question 2 : Calculer les coordonnées du point \(\displaystyle D\) tel que \(\displaystyle ABCD\) soit un carré.
Envoyer la proposition de travail
Lancer l'interactivité
On considère les points \(\displaystyle A\left(-1;4\right)\) , \(\displaystyle B\left(5;2\right)\) et \(\displaystyle M\left(x;y\right)\).
Question 1 : Calculer les coordonnées du milieu \(\displaystyle I\) de \(\displaystyle \left[AB\right]\).
Question 2 : Exprimer les coordonnées de \(\displaystyle \overrightarrow{MA}\), \(\displaystyle \overrightarrow{MB}\) et \(\displaystyle \overrightarrow{MI}\) en fonction de \(\displaystyle x\) et de \(\displaystyle y\).
Question 3 : Démontrer que \(\displaystyle \overrightarrow{MA}+\overrightarrow{MB}=2\overrightarrow{MI}\).
Envoyer la proposition de travail
Lancer l'interactivité
On considère les points \(\displaystyle A\left(-2;5\right)\) , \(\displaystyle B\left(2;-2\right)\) et \(\displaystyle C\left(6;3\right)\).
Question 1 : Déterminer les coordonnées du point \(\displaystyle D\), défini par \(\displaystyle \overrightarrow{AD}=\frac{3}{2}\overrightarrow{AC}\).
Question 2 : Déterminer les coordonnées du point \(\displaystyle E\), défini par \(\displaystyle \overrightarrow{BE}=\frac{-1}{8}\overrightarrow{BA}+\frac{3}{8}\overrightarrow{BC}\).
Question 3 : Démontrer que les points \(\displaystyle B\), \(\displaystyle D\) et \(\displaystyle E\) sont alignés.
Envoyer la proposition de travail
Lancer l'interactivité
On considère les points \(\displaystyle A\left(-2;-3\right)\) , \(\displaystyle B\left(3;3\right)\) et \(\displaystyle C\left(4;-1\right)\). L'origine du repère est le point \(\displaystyle O\) et on note \(\displaystyle I\) le milieu de \(\displaystyle \left[AC\right]\).
Question 1 : Calculer les coordonnées du point \(\displaystyle P\), défini par \(\displaystyle \overrightarrow{OP}=\overrightarrow{OA}-2\overrightarrow{OB}+\overrightarrow{OC}\).
Question 2 : Démontrer que les droites \(\displaystyle \left(OP\right)\) et \(\displaystyle \left(IB\right)\) sont parallèles.
Envoyer la proposition de travail
Lancer l'interactivité
Enoncé de l'exercice n° 8-7 : (Extrait : Exercice n°64 page 175 Déclic 2nde Hachette)
Dans un repère orthonormé \(\displaystyle \left(O;I;J\right)\) on considère les points \(\displaystyle A\left(-4;2\right)\), \(\displaystyle B\left(4;-6\right)\), \(\displaystyle C\left(8;6\right)\), \(\displaystyle D\left(3;1\right)\) et \(\displaystyle E\left(0;-2\right)\)
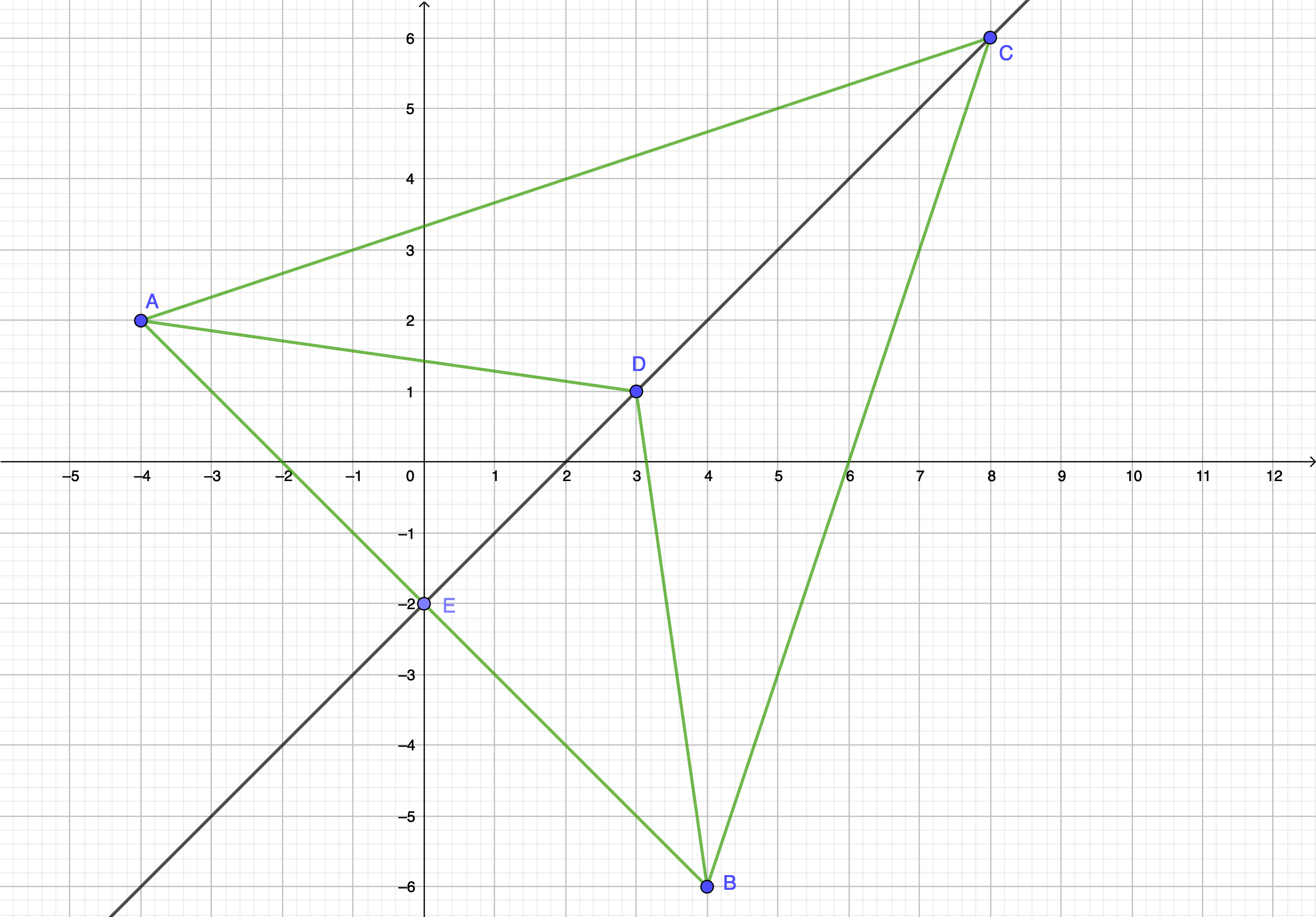
Question 1 : Montrer que le point \(\displaystyle D\) appartient à la médiatrice du segment \(\displaystyle \left[AB\right]\).
Question 2 : Déterminer la nature du triangle \(\displaystyle ABC\).
Question 3 : En déduire que \(\displaystyle \left(AB\right)\) et \(\displaystyle \left(CD\right)\) sont perpendiculaires.
Question 4 : Le point \(\displaystyle D\) est-il le milieu du segment \(\displaystyle \left[CE\right]\) ?
Question 5 : Calculer l'aire du triangle \(\displaystyle ABC\).
Correction de l'exercice n° 8-7 :
Question 1 : Pour montrer que le point \(\displaystyle D\) appartient à la médiatrice du segment \(\displaystyle \left[AB\right]\), on montre que \(\displaystyle AD=DB\) car tout point de la médiatrice est équidistant des points extrémités du segment.
Comme \(\displaystyle A\left(-4;2\right)\) et \(\displaystyle D\left(3;1\right)\) alors \(\displaystyle \overrightarrow{AD}\begin{pmatrix} 7 \\ -1\\ \end{pmatrix}\).
Comme \(\displaystyle D\left(3;1\right)\) et \(\displaystyle B\left(4;-6\right)\) alors \(\displaystyle \overrightarrow{DB}\begin{pmatrix} 1 \\ -7\\ \end{pmatrix}\).
Les normes de chaque vecteur donnent :
\(\displaystyle \begin{align*} AD&=\left\|\overrightarrow{AD}\right\| & DB&=\left\|\overrightarrow{DB}\right\|\\ AD&=\sqrt{7^2+(-1)^2} & DB&=\sqrt{1^2+(-7)^2} \\ AD&=\sqrt{49+1} & DB&=\sqrt{1+49} \\ &=\sqrt{50} & &=\sqrt{50} \\ \end{align*} \)
Comme \(\displaystyle AD=DB\), le point \(\displaystyle D\) appartient bien à la médiatrice.
Question 2 : Pour donner la nature du trianglen o calcule \(\displaystyle AB\), \(\displaystyle AC\) et \(\displaystyle BC\)pour vérifier l'équilatéralité :
Comme \(\displaystyle A\left(-4;2\right)\) et \(\displaystyle B\left(4;-6\right)\) alors \(\displaystyle \overrightarrow{AB}\begin{pmatrix} 8 \\ -8\\ \end{pmatrix}\).
Comme \(\displaystyle A\left(-4;2\right)\) et \(\displaystyle C\left(8;6\right)\) alors \(\displaystyle \overrightarrow{AC}\begin{pmatrix} 12 \\ 4\\ \end{pmatrix}\).
Comme \(\displaystyle B\left(4;-6\right)\) et \(\displaystyle C\left(8;6\right)\) alors \(\displaystyle \overrightarrow{BC}\begin{pmatrix} 4 \\ 12\\ \end{pmatrix}\).
\(\displaystyle \begin{align*} AB&=\left\|\overrightarrow{AB}\right\| & AC&=\left\|\overrightarrow{AC}\right\| & BC&=\left\|\overrightarrow{BC}\right\|\\ AB&=\sqrt{8^2+(-8)^2} & AC&=\sqrt{12^2+4^2} & BC&=\sqrt{4^2+12^2} \\ AB&=\sqrt{2\times 64} & AC&=\sqrt{144+16} & BC&=\sqrt{16+144}\\ AB&=8\sqrt{2} & AC&=4\sqrt{10} & BC&=4\sqrt{10}\\ \end{align*} \)
Comme \(\displaystyle AB\neq AC\) et que \(\displaystyle AC= BC\) , alors le triangle est isocèle.
On cherche ensuite à savoir si le triangle est rectangle ou pas. Le triangle étant isocèle, l'angle supposé droit ne peut être qu'au point \(\displaystyle C\).
\(\displaystyle \begin{align*} \text{On a d'une part } AC^2+BC^2&=\left(4\sqrt{10}\right)^2+\left(4\sqrt{10}\right)^2 & \text{ et d'autre part } AB^2&=\left(8\sqrt{2}\right)^2 \\ AC^2+BC^2&=220 & AB^2&=128 \end{align*} \)
On remarque que \(\displaystyle AC^2+BC^2\neq AB^2\), alors d'après la contraposé du théorème de Pythagore, le triangle n'est pas rectangle.
Question 3 : Comme \(\displaystyle AC= BC\), le point \(\displaystyle C\) est équidistant aux points \(\displaystyle A\) et \(\displaystyle B\). On en déduit que \(\displaystyle C\) appartient à la médiatrice de \(\displaystyle \left[AB\right]\). Les droites \(\displaystyle \left(AB\right)\) et \(\displaystyle \left(CD\right)\) sont donc perpendiculaires.
Question 4 : Le calcul des coordonnées du milieu de \(\displaystyle \left[CE\right]\) donne :
Comme \(\displaystyle E\left(0;-2\right)\) et \(\displaystyle C\left(8;6\right)\) alors les coordonnées du milieu sont \(\displaystyle \left(4;2\right)\). Elles ne sont pas égales à celles du point \(\displaystyle D\). Le point \(\displaystyle D\) n'est donc pas le milieu de \(\displaystyle \left[CE\right]\).
Question 5 : L'aire du triangle \(\displaystyle ABC\) se calcule par : \(\displaystyle A=\dfrac{AB\times EC}{2}\).
Comme \(\displaystyle E\left(0;-2\right)\) et \(\displaystyle C\left(8;6\right)\) alors \(\displaystyle \overrightarrow{EC}\begin{pmatrix} 8 \\ 8\\ \end{pmatrix}\).
\(\displaystyle \begin{align*} EC&=\left\|\overrightarrow{EC}\right\|\\ EC&=\sqrt{8^2+(8)^2} \\ EC&=\sqrt{2\times 64} \\ EC&=8\sqrt{2} \\ \end{align*} \)
\(\displaystyle \begin{align*} A&=\dfrac{AB\times EC}{2} \\ A&=\dfrac{8\sqrt{2}\times 8\sqrt{2}}{2} \\ A&=64 \\ \end{align*} \)
L'aire du triangle \(\displaystyle ABC\) est \(\displaystyle A=64\) u.a.
Envoyer la proposition de travail
Lancer l'interactivité
Enoncé de l'exercice n° 8-8 : (Extrait : Exercice n°84 page 178 Déclic 2nde Hachette)
Dans un repère orthonormé \(\displaystyle \left(O;I;J\right)\) on considère les points \(\displaystyle O\left(4;4\right)\), \(\displaystyle A\left(-1;4\right)\), \(\displaystyle B\left(8;7\right)\), \(\displaystyle C\left(0;1\right)\) , \(\displaystyle D\left(7;0\right)\) , \(\displaystyle T\left(1;8\right)\) et \(\displaystyle M\left(-7;2\right)\). La situation est résumée dans le schéma suivant :
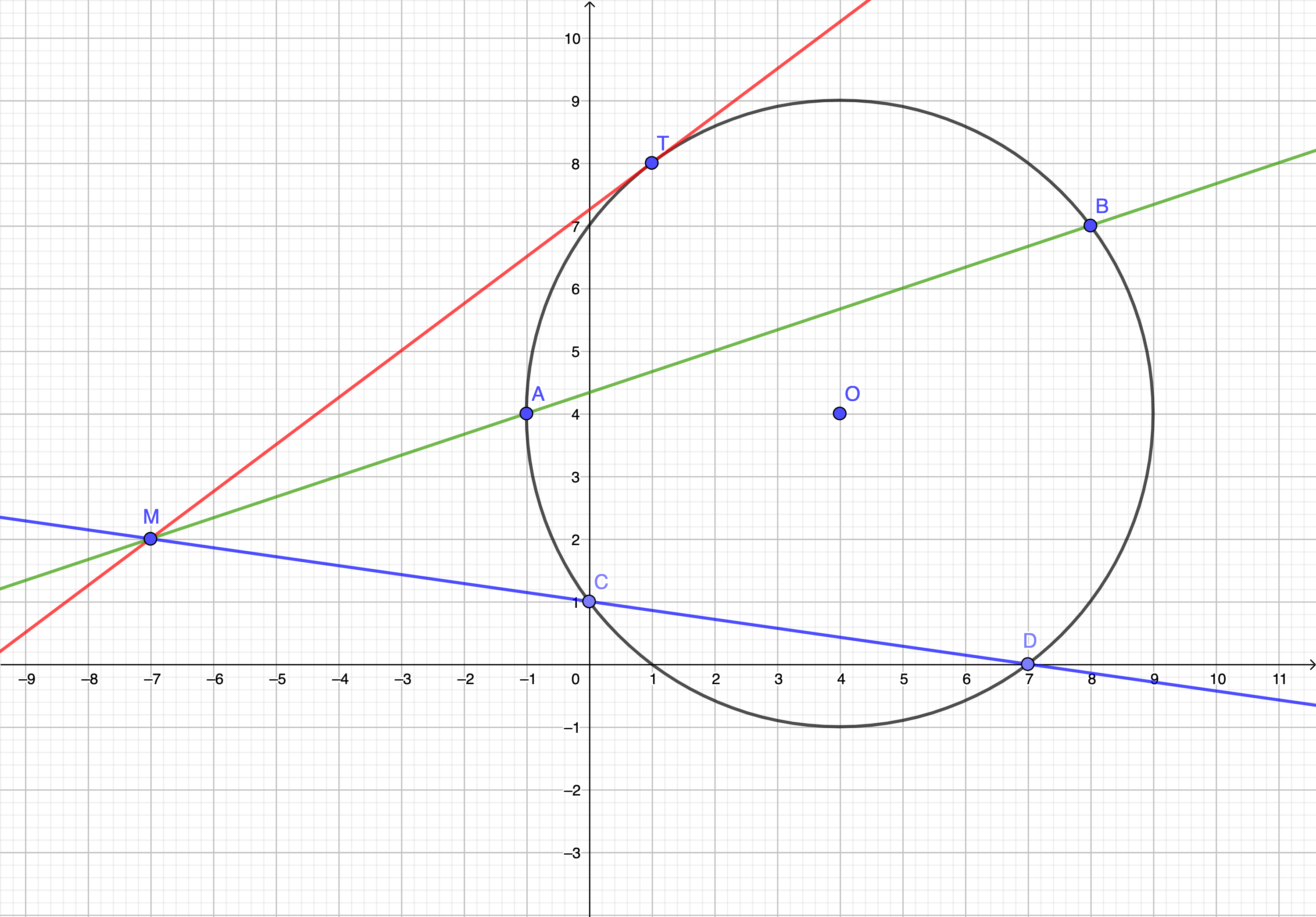
Question 1 : Justifier que les points \(\displaystyle A\), \(\displaystyle B\), \(\displaystyle C\), \(\displaystyle D\) et \(\displaystyle T\) appartiennent à un même cercle \(\displaystyle \mathcal{C}\) de centre \(\displaystyle O\).
Question 2 : Les points \(\displaystyle M\), \(\displaystyle A\) et \(\displaystyle B\) sont-ils alignés ?
Question 3 : Les points \(\displaystyle M\), \(\displaystyle C\) et \(\displaystyle D\) sont-ils alignés ?
Question 4 : Comparer les produits : \(\displaystyle MA\times MB\) ; \(\displaystyle MC\times MD\) et \(\displaystyle MT^2\).
Envoyer la proposition de travail
Lancer l'interactivité
Enoncé de l'exercice n° 8-9 : (Extrait : Exercice n°85 page 178 Déclic 2nde Hachette)
Dans un repère orthonormé \(\displaystyle \left(O;I;J\right)\) on considère les points \(\displaystyle A\left(2;4\right)\), \(\displaystyle B\left(-1;2\right)\) et \(\displaystyle C\left(6;-2\right)\).
On note \(\displaystyle \mathcal{C}\) le cercle circonscrit au triangle \(\displaystyle ABC\). La situation est résumée dans la figure suivante :
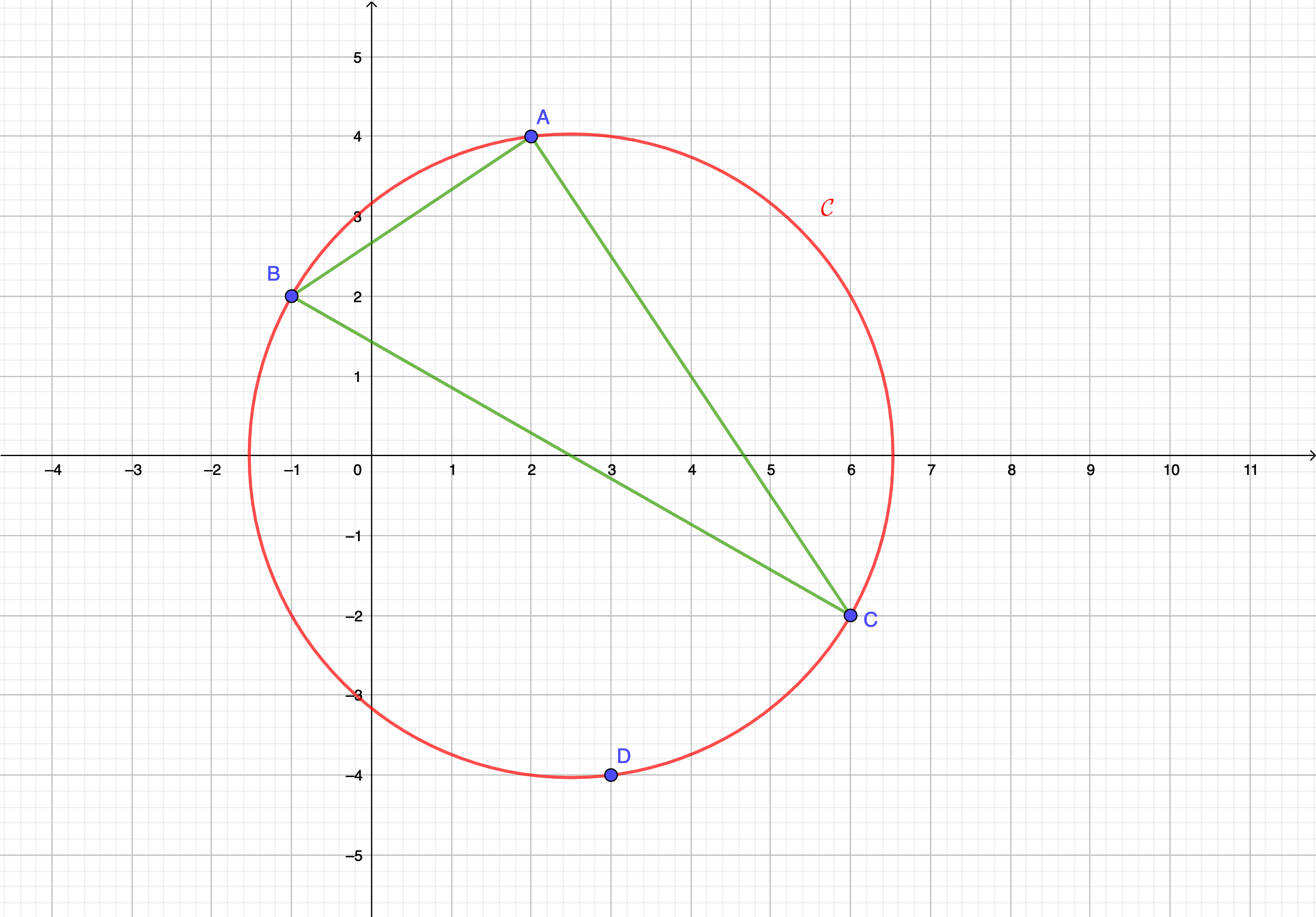
Question 1 : Donner la nature du triangle \(\displaystyle ABC\).
Question 2 : Déterminer le centre et le rayon du cercle \(\displaystyle \mathcal{C}\).
Question 3 : Justifier si le point \(\displaystyle D\left(3;-4\right)\) appartient au cercle \(\displaystyle \mathcal{C}\).
Question 4 : Déterminer les coordonnées des points \(\displaystyle E\) et \(\displaystyle F\) d'intersection de \(\displaystyle \mathcal{C}\) avec l'axe des abscisses.
Question 5 : Dire si le segment \(\displaystyle \left[EF\right]\) est un diamètre de \(\displaystyle \mathcal{C}\)
Envoyer la proposition de travail
Lancer l'interactivité